Einstein's Relativity
Albert Einstein published his first paper on relativity in 1905. It was entitled "Upon the Electrodynamics of Moving Bodies", which illustrates how Einstein's thinking about relativity grew out of the difficulties which physicists were having in reconciling James Clerk Maxwell's electromagnetic equations with Newtonian motion.Einstein was born in 1879. Unlike the young Isaac Newton – whose brilliance was obvious from an early age – the young Einstein was a lackadaisical dreamer. He was a mediocre student as a child, and he failed his college entrance exams and had to spend a year in a preparatory school before he finally got into the Federal Polytechnic Institute in Zurich. He graduated in 1900 with respectable but uneven grades, mostly because he cut nearly every class in the subjects that didn't interest him. (He had friends who let him study from their notes. Without them, it's doubtful he would ever have graduated.) Einstein much preferred lounging in coffee houses, and going sailing on Lake Zurich with his fiancée, than studying boring courses that did not use equations. After graduation, Einstein was unable to find a position as a scientific researcher or even as a high-school physics teacher, so he eventually accepted a position as Technical Expert, Third Class, in the Swiss patent office in Bern.
Einstein was later to say that his days in the Swiss Patent Office were the happiest of his life, because the work was easy and it left his evenings completely free so that he could think about physics with no outside intrusions.
What Einstein thought about, mostly, was the problem with electricity and magnetism. In particular, he kept coming back to the fact that Maxwell's equations are not Galilean invariant. This is a highly mathematical way of saying that if you do something (say, roll a ball across a table and watch it move in a straight line), will people who are moving with respect to you see the same thing if they repeat the experiment in their frame of reference? This depends. If they are riding smoothly in a railroad car moving in a straight line at constant speed, then the answer is yes. If they roll a ball on a table in the railroad car then it will behave exactly the same way as the one you rolled. Thus, the railroad car is said to be a Galilean invariant frame of motion.
However, if the people are on a rapidly spinning carousel, then the answer is no. The presence of centrifugal force will cause a rolling ball to veer sharply in a curving path away from the center of the carousel. Thus, the frame of motion of the carousel is said to violate Galilean invariance.
In this same spirit, mathematical equations can be analyzed with a technique called a "Galilean transformation", which tells us whether the form of the equations changes or not when shifted to another frame of motion. If the form doesn't change, then we have a case like that of the railroad car and the equations are called Galilean invariant. If the form does change, then we have a case like that of the carousel, and the equations are said to violate Galilean invariance. Maxwell's equations, when analyzed in this way, are not Galilean invariant, not even for a railroad car moving perfectly smoothly at constant velocity.
Victorian physicists circa 1880-1900 generally interpreted this as meaning that the ether (which was thought to fill space and served as the medium that light moved through) could more-or-less be considered as an absolute frame of reference, at least for electromagnetic phenomena. Like a fish in the ocean, maybe you didn't know your absolute position in that vast bulk of water, but you certainly knew whether or not you were swimming through it. The fact that Maxwell's equations violated Galilean invariance probably meant (they hypothesized) that it really did matter what your absolute velocity through the Universe was, since the ether had to form a cosmic ocean that could not possibly be at rest with respect to everything. This absolute motion had to be considered in addition to your velocity relative to the electromagnetic experiment you were doing. To put it another way, what if our friend inside that smoothly moving railroad car tried to duplicate his experiment with the rolling ball without any wind protection, so that the ball would be buffeted by 60 mph winds? This is analogous to how Victorian physicists thought about electromagnetism: it was taking place in a universal ether, just as things on Earth take place in a universal atmosphere, and yes, it definitely did matter whether you were standing still with respect to the ether, or rushing through it and experiencing a headwind.
Albert Einstein was decidedly underwhelmed by this logic. Quite aside from the fact that ether theories were compiling an utterly dismal record when it came to predicting anything real, Einstein was bothered by the whole concept of more-or-less tossing out Galilean relativity in favor of some hypothetical ether. In his writings, Einstein says that he eventually asked himself the question: What would a light wave look like, if I were traveling with it at the speed of light?
Well, Newtonian physics (i.e., the ether theories) said that the light wave would appear to be standing still. The electric and magnetic fields would oscillate before you, but go nowhere. This struck Einstein as total nonsense. There was nothing at all in Maxwell's equations which gave any indication that a stationary, oscillating field of this sort was possible, and indeed, the primary reason Maxwell had identified electromagnetic waves with light in the first place was because his equations predicted that the E & M waves would always move at the speed of light!
This paradox was known to other physicists, and it was one reason that many theoretical physicists felt that Maxwell's equations were incomplete in some way, or maybe simply wrong. Despite the success of Maxwell's theory, creating alternative electromagnetic theories was practically a small industry towards the end of the 19th
Perhaps fortunately, Einstein was not a physics professor. His position in the Swiss Patent Office did not provide Einstein with much access to the latest scientific literature, so his thoughts about electromagnetic theory were occurring in something of a vacuum. There were no older colleagues on hand to immediately contradict him and bury him under complex ether arguments when he eventually decided that it was Newton's physics which would have to go, and not Maxwell's.
Einstein realized that Maxwell's equations not only predicted that electromagnetic waves would move at the speed of light, but also that their speed would be
Both k and m are fundamental constants, like the gravitational constant G or Boltzmann's constant kB. They never change. Thus, the speed of light is just a number in Maxwell's theory, with no dependence on anything. c is exactly like the number of fingers on your hand: five is five, regardless of anything that has to do with motion. Einstein's most critical insight was probably his realization that there actually wasn't anything in Maxwell's theory which said that this constant speed only applied if you were at rest with respect to the light source. Everybody in the physics world assumed it did, everybody felt free to add or subtract velocities to light-speed for moving light sources in the traditional Galilean
He concluded that the speed of light is a constant, to all observers, under all circumstances.
That statement is so simple, yet hides such complexities, that it deserves to be restated.
The speed of light in a vacuum is the same for all observers.
This statement is much stranger than it looks. Let's suppose you are standing beside a highway and see a car approaching at 60 miles per hour: its speed relative to you is 60 mph. Now suppose you start your car and drive towards the other car at 50 mph. The relative speed of the other car is now 50 + 60, or 110 mph. This is in accordance with common sense and Newtonian physics.
But in Einstein's relativity, this kind of simple addition cannot be true. If I time the light coming from a stationary source, I will
No. I will still measure exactly 186,282 miles per second as the light races past me, exactly as before, because:
The speed of light in a vacuum is the same for all observers.
This statement implies some things that violate common sense with a vengeance. Einstein knew this, and his explanation was: speed is defined as distance divided by time. In Newtonian physics, one assumes that a meter is a meter, and a second is a second. Time and space are the same for everyone. But when you say that a certain speed, which is a distance divided by time, must be the same for all observers no matter their relative velocity to each
And with that, Newtonian physics crashes to the ground. The absolute constancy of the speed of light in a vacuum is the fundamental postulate of Einstein's relativity. Modern physicists consider this postulate to be one of the fundamental physical laws of the
In his paper in 1905, Einstein pointed out this logic, then proceeded to calculate exactly what form this transformation of time and space would take. The crucial point was, would the calculations lead to some obvious absurdity that could be proven wrong with an experiment? Or would they, in fact, begin to explain some things that were previously unexplained? Let's discuss what two of the (simpler) predictions were.
1) Length contraction. Let's say you measure the length of your car. Then, you have a friend drive your car past you. You measure its length again as it is moving by (with a high-tech laser gizmo). You will find that the measured length of your car when it is moving is less than the measured length when it is standing still with respect to you.
I did not say that the car had been "crushed" in some way. I said its length was less. The length contraction is an actual contraction of the space occupied by the car, from your point of view. However, from the viewpoint of a passenger inside the car, there is no change and the length of the car remains exactly the same! Which length is "real", you ask?
Answer: both are, because one of you is moving with respect to the car, and one isn't. In Newtonian physics this fine distinction is meaningless, because length is considered absolute and independent of relative velocity. In Einstein's physics, the distinction is very important. Since the car is standing still with respect to the passenger, she will measure its length to be exactly the same as you did when the car was standing still with respect to you. (The invariant length that someone at rest relative to the car will always measure is called the car's rest length. If the car has a "real" length, this is it.) But anyone moving with respect to the car will always measure a different, shorter length as the car goes by.
In the illustration below, you can see exactly how the velocity of a passing starship changes its length in your rest frame. In the rest frame of the ship, of course, the ship is completely unchanged and YOU are the one undergoing length contraction!
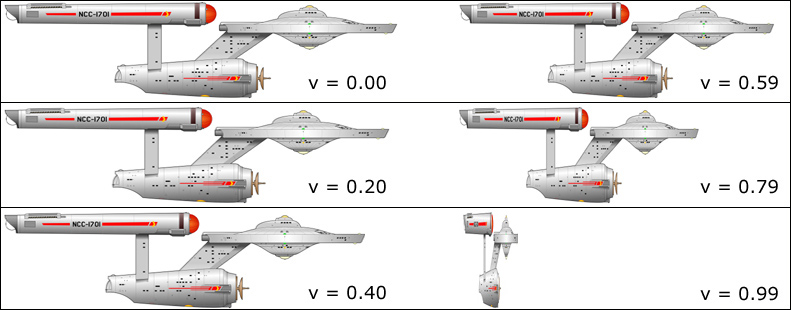
The Starship Enterprise, as it approaches the speed of light. (Velocity is given as a fraction of lightspeed.) Note that the height of the starship does not change, only its length. Also, note how nearly imperceptible the contraction is even at 20% of lightspeed, and it isn't large even at 59%. Relativistic effects generally do not become large until you are very close to the speed of light.
2) Time dilation. Let's suppose that you and your friend synchronized watches just before she got in the car. They are identical, and keep perfect time. As your friend motors by, you observe her watch with a telescope and compare it with your own. You find that her watch is now running more slowly than yours. However, your friend (who has a telescope in the car and is watching your watch) finds that her watch is the one running perfectly, and your watch is the one that's running more slowly! In answer to the inevitable question, "Which watch is really running the most slowly?", all I can say is: what do you mean by "really"? From whose point of view? In Einstein's physics, both points of view are equally valid because time itself (like length) is relative to the observer.
These predictions that an object moving with respect to you will contract in space and experience a slower time flow (from your point of view, not from the object's point of view) sound
This is where mathematics comes in. The formulas on the relativity handout clearly show what happens in relativity when the relative velocities are small compared to the speed of light. When v is some paltry speed like 30 m/s (67 mph), the space occupied by a moving car will contract by only 0.0000000000005%. This represents something like a thousandth of the radius of a hydrogen atom. A contraction that small is not exactly something you notice with the naked eye.
The relativistic formulas for time dilation, mass dilation, etc. similarly yield extremely small numbers for velocities that are small compared to c. Relativity has not replaced Newtonian mechanics so much as it has proved that Newtonian mechanics is a real, real, real good approximation to relativity for "small" velocities like 1000 miles/second. Einstein's original paper pointed this out, and (in effect) said that the theory cannot be dismissed just because it seems counter to common sense. Common sense is based on very slow speeds, but relativity comes into play mostly at very high speeds.
It is tempting to think of relativistic effects as only illusions or as things that just look a certain way depending on your speed. Not true. For example: the muon is an unstable subatomic particle that has an average lifetime of 2.2 X 10-6 sec. Despite this, muons can be readily detected at the Earth's
The problem is, even if the muons are moving at very nearly the speed of light, they can still only travel an average of
The answer is, the very high-energy atomic collisions which produce the muons also propel them at terrific velocities, typically faster than 99.9% the speed of light. This is so fast that the relativistic time-dilation effect slows down their disintegration time to the point where they can easily reach the Earth's surface. This is no mere illusion. With relativistic time dilation, the experiment in Phyx 359-3 can be done in Tech. Without it, we'd have to float the apparatus to 25,000 ft on a weather balloon.
In addition to the formulas for length and time, relativity changes everything else associated with motion, including mass, energy, momentum, and force. Similar to the relativistic behavior for length and time, the relativistic behaviors of mass, force, etc. are indistinguishable from their Newtonian cousins when the speed is more like that of a high-power rifle bullet, rather than millions of meters per second. Classic Newtonian physics works fine for "low" velocities. It is only when one approaches a significant fraction of the speed of light that things begin to get strange.
Which brings us to the Michelson-Morley experiment of 1887. They had tried to detect differences in the speed of light as the Earth changes its speed relative to the ether (which it must, since it orbits the Sun in a circle). But to their great surprise, they found that the speed of light never changed, regardless of where they looked or how they made the measurement.
This is very strange from the viewpoint of Newtonian physics. Victorian physicists did many experiments aimed at trying to figure out what the ether was
The meaning of most of the other ether experiments fell into place too, once they were analyzed from the viewpoint of relativity. The experiment on the speed of light through moving water was a particular triumph for relativity (see the
Einstein made a number of predictions about experiments which could be used to test relativity (which have since been carried out, and do indeed validate the
Classic Relativity ParadoxThe rules of relativity truly play havoc with your intuition, when speeds get high enough. As an example, let's consider a relativity classic, the rocket in a barn problem.
Suppose we have a rocket of rest length Lo, roaring very relativistically towards a barn with the same rest length Lo. (The top figure shows the rocket at rest with respect to the barn, or alternatively, it shows the length of the rocket at any velocity as it roars past the barn according to classical Newtonian physics.) The barn has doors at either side that we'll call A and B. They open automatically when the rocket reaches them, and close automatically after the rocket passes.
Let's start by supposing that we are in the barn. For an observer in the barn's rest frame, the rocket is highly length-contracted as it passes. That is, the rocket can easily fit into the barn, as shown in the middle figure at right. So, an observer in the barn will see the following sequence of events:
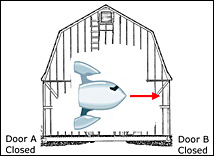
- Squashed rocket reaches barn
- Front of rocket reaches Door A, door opens (Event A1)
- Tail of rocket leaves Door A, door closes (Event A2)
- Squashed rocket is entirely inside barn, as shown in the middle figure.
- Front of rocket reaches Door B, door opens (Event B1)
- Tail of rocket leaves Door B, door closes (Event B2)
- Squashed rocket leaves barn behind
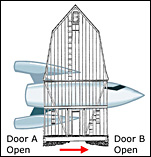
- Rocket reaches squashed barn
- Front of rocket reaches Door A, door opens (Event A1)
- Front of rocket reaches Door B, door opens (Event B1)
- Rocket is sticking out both sides of squashed barn, as shown in the bottom figure.
- Tail of rocket leaves Door A, door closes (Event A2)
- Tail of rocket leaves Door B, door closes (Event B2)
- Rocket leaves squashed barn behind
Well, no, actually, it doesn't. When you start stretching time and space, odd things can happen besides a few things getting a little shorter. The key point about the rocket in a barn problem that you must focus on is the exact order in which events happen in both frames, and where they are happening. Events that occur at the same point in space (even if that point is moving) will always obey the same ordering that Newtonian physics expects them to. Events that occur at different points in space?
For example, regardless of your frame of motion, you will always see Door A open before it closes (Event A1 always precedes Event A2). These two events occur at the same point in space, and therefore cannot be reversed in order. Similarly, Door B will always open before it closes (Event B1 precedes B2). Also, Door A will always open before Door B in any frame of motion (Event A1 precedes Event B1), because both events are initiated by the same point in space: the front of the rocket. The fact that this point is moving is neither here nor there, it is still a single point. Likewise, Door A will always close before Door B (Event A2 precedes Event B2) because the tail of the rocket is a single point.
Let us examine the two lists of events just above. As predicted, Event A1 is before Event A2 in both frames, and B1 is also before B2 in both. In other words, the doors opened before they closed. A1 is before B1 in both, and A2 is before B2 in both, which means that the front of the rocket always arrived before the tail. So far, so good. But take a close look at Events B1 and A2: they are reversed in the two frames!? Is that right? Can you really move things around in time like that?
You betcha. The critical thing about Events B1 and A2 is that they do NOT have a single point in space in common. The tail of the rocket is at a different point in space than the front of the rocket, and the two doors are in different places. Thus, the point in time when the tail of the rocket causes Door A to close, and the point in time when the front of the rocket causes Door B to open, are not related. There is no strictly logical reason why the second door must open either before or after the first door closes, and as demonstrated above, it may do either, depending on where you are and how fast you are moving. This reversal of events in time is exactly what places the rocket inside the barn in one frame, but sticking out both ends in the other.
The fun physics here is that words like "before" and "after" mean one thing when you have absolute time (Newtonian physics), but something else entirely when you have relative time. Newtonian physics lets you be very "sloppy" when it comes to distinguishing between what must happen before something else, and what only happens to happen before something
Completely unrelated to the bank robbery. In a relativistic universe, that means an observer might see the robbery at six, or before six, or after six, depending on where they are and how fast they are moving. In Newtonian physics, things that must happen in a certain order, and things which just happen to happen in a certain order, are equally invariant. This is not true with relativity, which explains "paradoxes" such as the rocket in a barn problem.
To put it another way, you will always see Sammy Sosa hit the baseball before it leaves the park, either classically or relativistically. The question is, what about that guy down in the bullpen, with his back to the play? Did he throw his practice pitch before, or after, Sosa swung his bat? With Newton, you will always get the same answer for all observers. For
E = mc2
The famous equation E = mc2 is what comes out of relativity when you look at what has to happen to energy, momentum, and force for objects moving near the speed of light. This equation tells us that, in addition to all its other myriad forms (mechanical, chemical, thermal, gravitational, etc), energy can also appear as mass! Therefore, the principle of conservation of energy must be replaced by the more general principle of conservation of mass-energy. Energy and mass are the same thing, except for a constant factor.
One does not normally notice mass changing into energy, or vice versa, for the simple reason that c2 is enormous, about 1017. It takes 9.8 joules of energy to lift a one-kilogram weight one meter, and when that energy changes into mass, it represents an increase of only 0.00000000000001% for the mass of the kilogram weight. The amounts of energy that one encounters in day-to-day life are not nearly large enough to create noticeable mass-conversion effects; for that, one must go to very high velocities and very high energies.
It is important to understand how literal the mass-energy relationship is. Since energy is equivalent to mass, that means energy is attracted by gravitational fields, and generates a gravitational field of its own. When you shine a flashlight across the room, the beam of light begins falling with a constant acceleration of 9.8 m/s2 just as any mass does. Light and all other forms of energy can carry momentum, and can exert forces.
When you heat up a pot of spaghetti sauce, the pot becomes more massive, because you've added thermal energy to it. When you lift a kilogram weight and give it "gravitational potential energy", you've made it more massive. What you should realize is that the rather vague concept of "gravitational potential energy" (or "chemical potential energy", or "elastic potential energy", etc) is very Newtonian. In this view, energy is energy and matter is matter, so energy is something that is moved here and there and somehow "added" to matter in the form of various potential energies. In relativity, mass and energy are equivalent and when you say that something has gained energy (of the potential variety or otherwise), you also mean that it has gained mass.
Relativity modifies all of the Newtonian equations for force and motion, including F = ma, p = mv, and K.E. = ½ mv2. F and p are easy to modify: you just have to replace "m" with
E = mc2 refers to the total energy of a body, that is, both any kinetic energy (and/or chemical, and/or thermal, etc) which the object possesses, plus the equivalent of converting its entire rest mass into energy. The "m" in E = mc2 is not the rest mass of an object, but rather the dilated mass,
K.E. = mc2 - moc2 = moc2/ (1 - v2/c2)½ - moc2
Some simple algebraic rearrangement yields the expression for relativistic K.E. given in the handout.
The Ultimate Speed Limit
The relativistic equations for length, mass, time, force, energy, and momentum all contain the factor
The flip side of this is that any massless entity (light, gravity waves, various exotic creations from the world of particle physics) must move at the speed of light. No faster and no slower. It is simply not possible for pure energy with no rest mass to materialize below the speed of light. The Universe only recognizes two ways to transport energy and momentum: with massive particles traveling below the speed of light, or with massless energy traveling exactly at the speed of light.
Experimental Tests
Historically, it took some time after 1905 before physicists had the means to directly test relativity. At first, the experimental verifications of relativity were simply that it could easily explain phenomena that the ether theories could not explain. I have already mentioned the Michelson-Morley experiment and the experiment on lightspeed through flowing water.
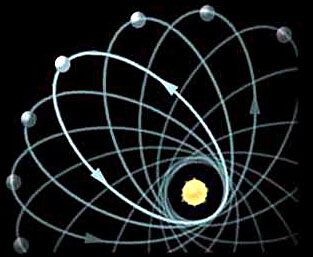One famous calculation that Einstein carried out shortly after his publication of the theory concerned the precession of the perihelion of Mercury. The planets move in elliptical orbits around the Sun, and in the normal course of things the orientation of those orbits do not change, due to the conservation of angular momentum. But astronomers in the mid-19th century had discovered that Mercury was different. Its elliptical axis slowly shifted with every orbit. (The figure at left illustrates the basic idea, but the degree of precession is extremely exaggerated.) In Newtonian physics, this could only happen if something were exerting a force on Mercury, so astronomers postulated that there must an unknown planet, very small and orbiting very close to the Sun, that was pulling on Mercury. The new planet was christened Vulcan, after the Roman god of the forge, and it was assumed that no one had seen it before because it was always lost in the glare of the Sun.The trouble was, 60 years had gone by and still no one had seen Vulcan, despite some very determined tries. And when some physicists tried to use Mercury's positions to calculate backwards and deduce where Vulcan would have to be, they got very odd, inconsistent results.
Einstein pointed out that Mercury is the innermost planet, and therefore the fastest moving. At its closest approach to the Sun, Mercury is moving at about 32 miles/sec. This is far below the speed of light, but it is just enough to relativistically dilate Mercury's mass so that its momentum slightly increases just enough to account for the observed precession. Einstein calculated the exact amount of precession that would result if relativity were correct, and the calculation agreed well with observations.
More direct tests of relativity had to wait for better technology. I have already discussed the obvious effect of time dilation on the average lifetime of the muon; this experiment was first carried out in 1941. In 1964, a particle accelerator was used to send an extremely high-energy beam of pions (another unstable subatomic particle) across a laboratory at 99.975% of the speed of light. When pions disintegrate they emit gamma-rays, which move at lightspeed. The speed of the disintegration gamma-rays were measured, and they were in fact found to be moving at exactly the speed of light, even though they were launched from a platform that was itself moving at virtually the speed of light with respect to the laboratory. In other words, they proved that:
In 1977, two extremely high-precision clocks (accurate to something like one part in 1014) were synchronized, then one was loaded onto a jet aircraft while the other stayed on the ground. After the aircraft had circled the world, the two clocks were compared and - by golly. They were out of synch. Time dilation had caused the clock aboard the aircraft to slow down and lose an infinitesimal fraction of a picosecond as compared to the clock left on the ground.
Today, relativity is one of the bedrocks of modern physics. The field of particle and nuclear physics could not exist without it, as well as large swaths of astrophysics and plasma physics. Relativity is proven every day in the pages of physics journals, where authors writing about everything from solar storms to high-precision electron spectroscopy describe their results using physics which would make no sense at all and give erroneous results if relativity were not true.
Relativity is now over 100 years old and is embedded into modern physics as thoroughly as Newton's Laws of Motion. It is probably time we stopped calling it a theory, and promoted it to a Principle.
Electricity & Magnetism
|
Quantum Mechanics Timeline 
Ideas of Physics Homepage
A Ukrainian translation of this page is available here: Ukrainian